Our Simulators
Interactive Simulators for Hands-On Science Exploration
Interactive Simulators for Hands-On Science Exploration
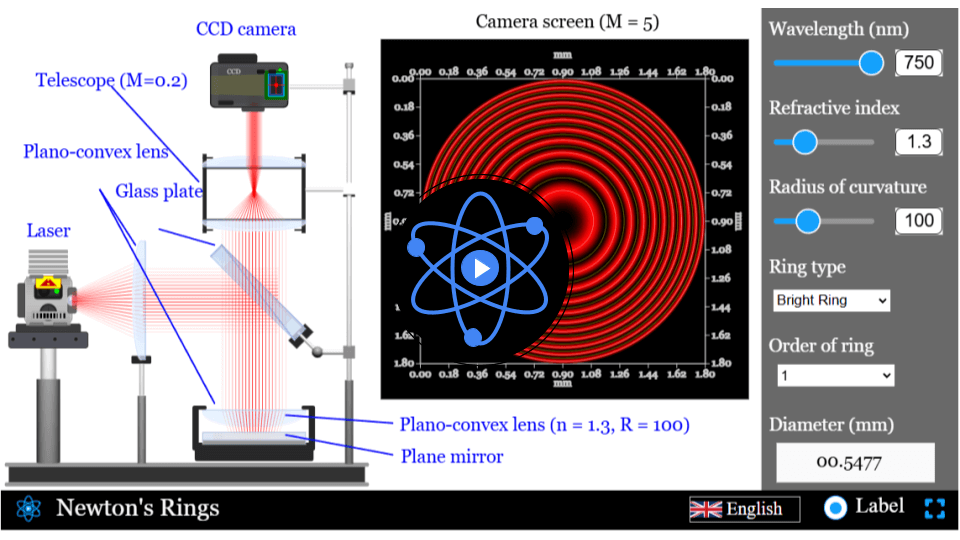
Physics surrounds us, influencing countless aspects of our daily lives, often in ways we overlook. Have you ever noticed the...
Read More
Few discoveries in physics have impacted our understanding as deeply as the phenomenon of single-slit diffraction...
Read More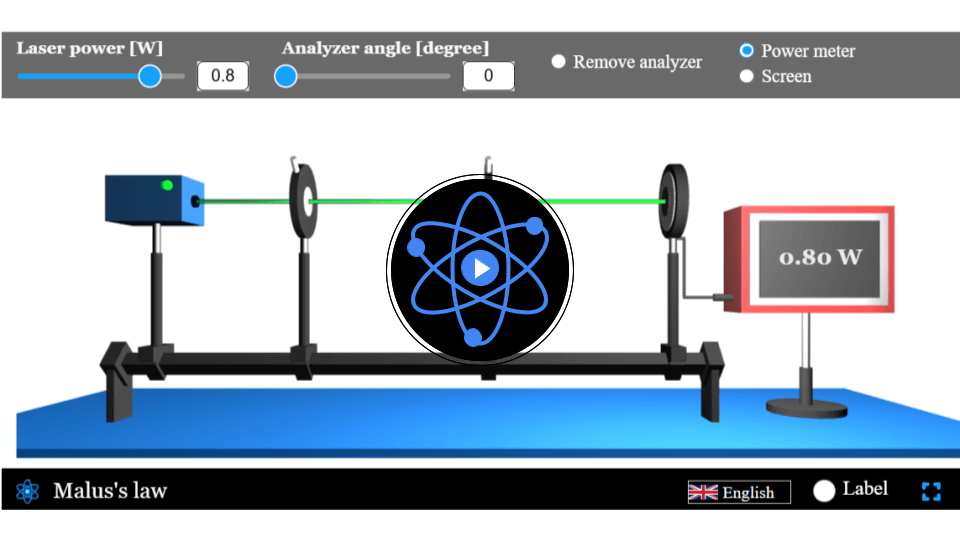
Physics is woven into our everyday world, influencing how we perceive and interact with light. Have you ever wondered...
Read More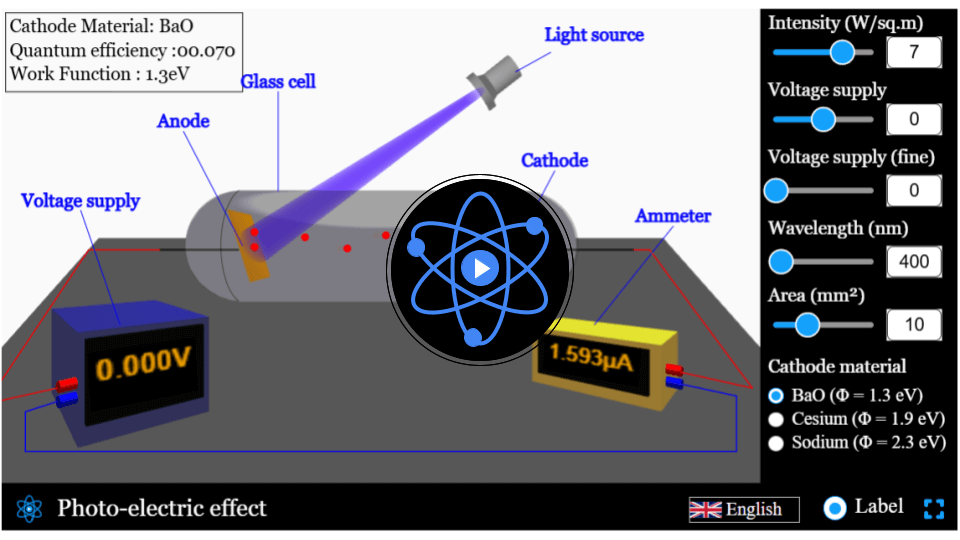
Physics is all around us, shaping our everyday experiences in ways we might not even notice. Have you ever wondered...
Read MoreDiscover interactive science simulators at Explerify.com! Enhance classroom learning with hands-on tools designed to make science fun and engaging.
We aim to make virtual experimental labs accessible to all educational institutions.
* Available on all devices, from projectors to handhelds.

"As a science outreach volunteer, I'm always looking for engaging and accessible tools to inspire curiosity in students—and this website is a true gem! It offers beautifully designed, interactive simulations of physics experiments...."
"This website is a brilliant and engaging resource for anyone exploring Physics. It presents concepts in a highly creative and visual way, making it much easier to understand complex topics—whether you're learning about light, atoms, or electric charges...."
"Really helpful and supportive domain for high school and undergraduate students inclined towards building an intuitive understanding towards various concepts in Physics...."